Coupled Potentials
A series of coupled chemical and electrochemical potential gradients power the cell (Figure 1). There is a constant flux of glucose and oxygen into neurons and most other cells. Oxidation of glucose is coupled to the generation of an ion gradient, the proton gradient across the inner wall of the mitochondria. This ion gradient is coupled to the generation of a large ATP/ADP gradient. The ATP/ADP gradient in the cytoplasm is, in turn, coupled to the generation of ion gradients across the cell membrane. The cell membrane sodium gradient is coupled to the transport of a myriad of solutes as well as the generation of an electric potential gradient. Energy is readily interconverted between electrochemical potentials associated with ion gradients and chemical potentials associated with chemical reactions.
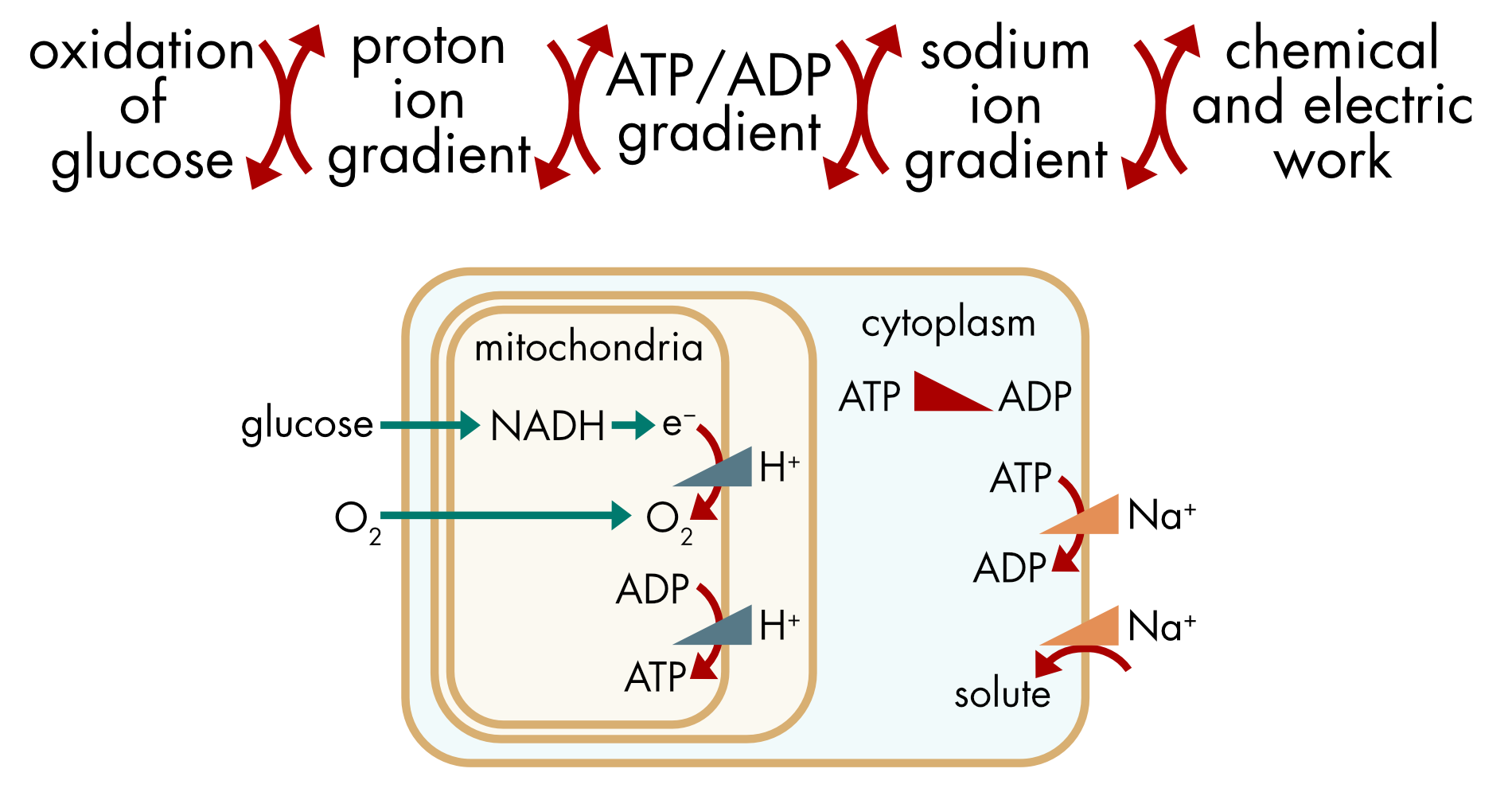
Figure 1 Coupled chemical and electrochemical potentials. The ATP/ADP and the sodium ion gradients are coupled to multiple processes in the cell that perform the chemical and electric work necessary for the maintenance of cellular life.
The stoichiometry of the various pumps and transporters involved in these different processes can seem quite arbitrary. Some pumps move a single ion while others move five. Similarly, transporters move different numbers of solutes. In this section we will see that these stoichiometries are strictly constrained by the chemical potentials of the coupled processes. The driving force provided by the energetically favorable process has to match or exceed the potential gradient of the energetically unfavorable process in order for the pump or transporter to function. The magnitude of the potential gradient up which a given solute has to be transported can differ between different compartments or cells. This explains why almost identical pumps or transporters can have different stoichiometries.
Pumps
Na,K-ATPase
The Na,K-ATPase couples vectorial movement of sodium and potassium ions to the hydrolysis of ATP. This coupled reaction can be written as,
\[\text{3N}\text{a}_{\text{in}}^{\text{+}}\text{\ +\ 2}\text{K}_{\text{out}}^{\text{+}}\text{\ +\ ATP\ ⇀\ 3N}\text{a}_{\text{out}}^{\text{+}}\text{\ +\ 2}\text{K}_{\text{in}}^{\text{+}}\text{\ +\ ADP\ +}\text{P}_{\text{i}}\]
In a cell with \(\left\lbrack \text{Na} \right\rbrack_{i} = 15\) mM, \(\left\lbrack \text{Na} \right\rbrack_{o} = 140\) mM, \(\left\lbrack \text{K} \right\rbrack_{\text{i}}\text{=130}\) mM, \(\left\lbrack \text{K} \right\rbrack_{\text{o}}\text{=4.5}\) mM and \(V_{m} = - 0.07\ V\) (-70 mV), the electrochemical potential difference for sodium ions is:
\[\begin{aligned}\Delta{\widetilde{\mu}}_{\mathit{Na}} &= \text{RT\ ln}\frac{\left\lbrack \text{Na} \right\rbrack_{i}}{\left\lbrack \text{Na} \right\rbrack_{o}} + z\text{F}V_{m}\\\\[-2ex] &= - 5,760 - 6,754\ \text{J}\text{⋅}\text{mo}\text{l}^{\text{-1}}\\\\[-2ex] &= - 12.5\ \text{kJ}\text{⋅}\text{mo}\text{l}^{\text{-1}}\end{aligned}\]
The sign has to be reversed because sodium ions are moved out the cell by the pump. The electrochemical potential difference for potassium ions is considerably smaller:
\[\begin{aligned}\Delta{\widetilde{\mu}}_{K} &= \text{RT\ ln}\frac{\left\lbrack K \right\rbrack_{i}}{\left\lbrack K \right\rbrack_{o}} + z\text{F}V_{m}\\\\[-2ex] &= 8,673 - 6,754\ \text{J}\text{⋅}\text{mo}\text{l}^{\text{-1}}\\\\[-2ex] &= 1.9\ \text{kJ}\text{⋅}\text{mo}\text{l}^{\text{-1}}\end{aligned}\]
The Na,K-ATPase moves five ions across the cell membrane for the expenditure of one ATP molecule. The total potential gradient for the movement of five ions is given by,
\[\begin{aligned}3\ \Delta{\widetilde{\mu}}_{\mathit{Na}} + 2\ \Delta{\widetilde{\mu}}_{K} &= 3 \times 12.5 + 2 \times 1.9\\\\[-2ex] &= 41.4\ \text{kJ}\text{⋅}\text{mo}\text{l}^{\text{-1}}\end{aligned}\]
The chemical potential difference for ATP hydrolysis is more than -50 kJ⋅mol-1 in a healthy cell, so the hydrolysis of ATP provides sufficient energy to move these five ions up their concentration gradients, as expected. This process would be likely to fail however if the pump were coupled to the movement of four sodium ions instead of three. The amount of energy that is available limits the stoichiometry of the pump.
Ca2+-ATPase
Two different calcium pumps are found in the plasma membrane and the membrane of the endoplasmic or sarcoplasmic reticulum (Figure 2). They are both P-type ATPase pumps and have similar structures. The pump found in the plasma membrane is known as PMCA (Plasma Membrane Ca2+-ATPase). The pump found in the SR/ER membrane is known as SERCA (Sarco/Endoplasmic Reticulum Ca2+-ATPase). The two pumps differ in an important way. The PMCA moves one calcium ion for the expenditure of one ATP molecule whereas SERCA moves two calcium ions. It is worthwhile understanding the reason for this difference.
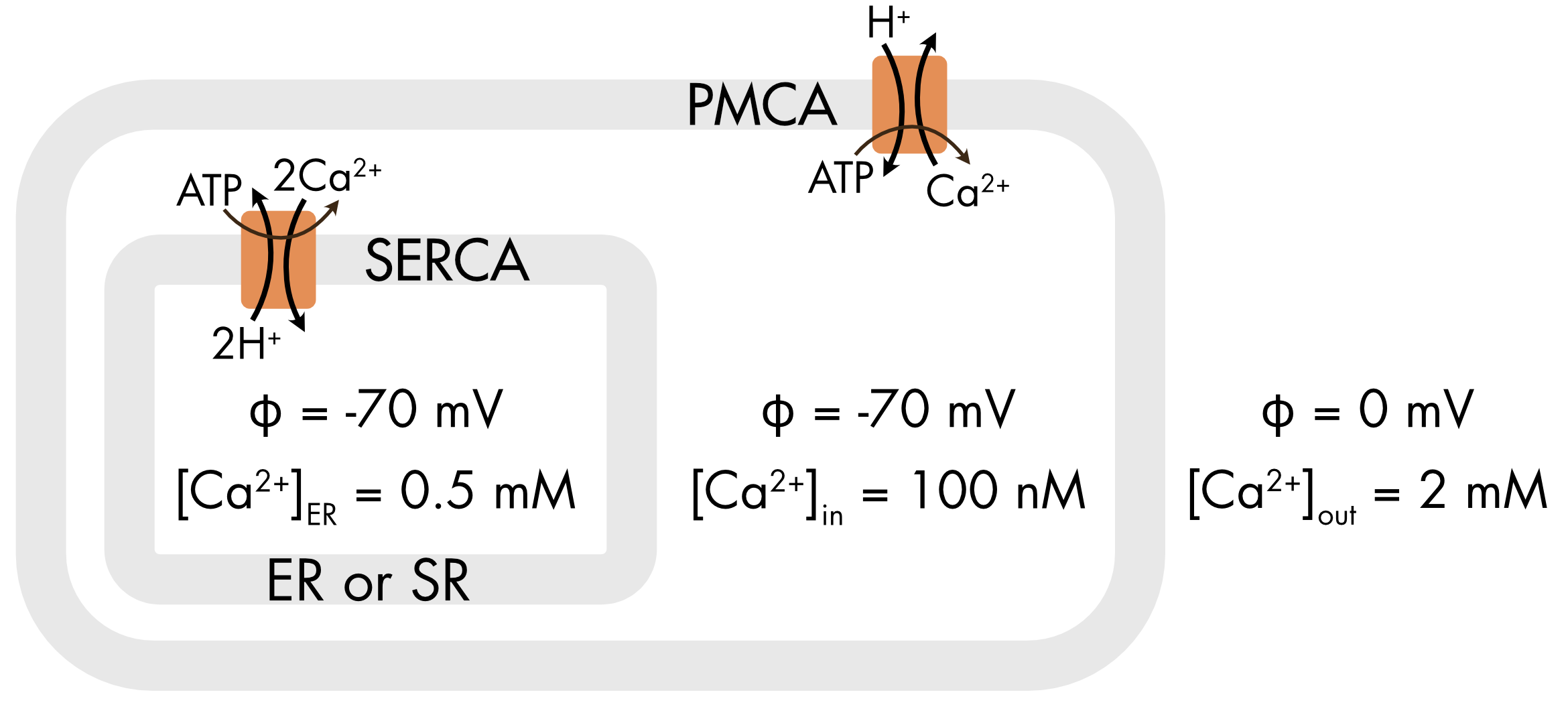
Figure 2 The PMCA and SERCA Ca2+ pumps differ in the number of calcium ions coupled to the hydrolysis of one ATP molecule.
For the PMCA, the coupling of ion movement to hydrolysis of ATP can be written as,
\[\text{C}\text{a}_{\text{in}}^{\text{2}\text{+}}\text{\ +\ ATP\ ⇀\ C}\text{a}_{\text{out}}^{\text{2}\text{+}}\text{\ +\ ADP\ +\ }\text{P}_{\text{i}}\]
Calculation of the electrochemical potential difference for calcium ions between the cytoplasm and the extracellular solution reveals why the PMCA moves only a single calcium ion.
\[\begin{aligned}\Delta{\widetilde{\mu}}_{\text{Ca}} &= \text{RT\ ln}\frac{\left\lbrack \text{Ca} \right\rbrack_{\text{in}}}{\left\lbrack \text{Ca} \right\rbrack_{\text{out}}} + z\text{F}V_{m}\\\\[-2ex] &= - 25,538\ - 13,508\ \\\\[-2ex] &= - 39.0\ \text{kJ}\text{⋅}\text{mo}\text{l}^{\text{-1}}\end{aligned}\]
The sign has to be reversed because calcium ions are moved out the cell by the pump. ATP hydrolysis provides sufficient energy (-50 kJ⋅mol-1) for the transfer of only a single calcium ion across the cell membrane.
For the SERCA, the coupling of ion movement to hydrolysis of ATP can be written as,
\[\text{2C}\text{a}_{\text{in}}^{\text{2}\text{+}}\text{\ +\ ATP\ ⇀\ 2C}\text{a}_{\text{ER}}^{\text{2}\text{+}}\text{\ +\ ADP\ +\ }\text{P}_{\text{i}}\]
There is little or no electric potential difference across the ER membrane and the gradient for calcium ions is lower (Figure 2). As a consequence, the electrochemical potential difference for calcium ions across this membrane is significantly lower than across the plasma membrane.
\[\begin{aligned}\Delta{\widetilde{\mu}}_{\text{Ca}} &= \text{RT\ ln}\frac{\left\lbrack \text{Ca} \right\rbrack_{\text{in}}}{\left\lbrack \text{Ca} \right\rbrack_{\text{ER}}} + z\text{F}V_{m}\\\\[-2ex] &= - 21,964 - 0\ \ \\\\[-2ex] &= - 22.0\ \text{kJ}\text{⋅}\text{mo}\text{l}^{\text{-1}}\end{aligned}\]
ATP hydrolysis (-50 kJ⋅mol-1) provides sufficient energy to move two calcium ions (43.9 kJ⋅mol-1) into the ER with each pump cycle.
The difference in the electrochemical potential for calcium ions across the cell membrane compared to the SR/ER membrane accounts for the different stoichiometries of these two pumps, which are otherwise performing an identical task.
Calcium pumps also transport protons as a counterion, but proton coupling contributes little to the energy budget.
Transporters
Pumps are essentially irreversible; the ATPase of the pump is ineffective at catalyzing the reverse reaction even if thermodynamics favor reversal. Transporters on the other hand are easily reversed. The direction of transport depends only on the balance of electrochemical and chemical potentials of the transported ions and solutes. The concentration of these ions and solutes as well as the membrane potential can vary over time or between different cells or cellular compartments resulting in reversal, which can pose problems for the organism.
Na/Glucose Transporter
Movement of glucose by the Na/glucose transporter depends on the electrochemical potential of the sodium ions coupled to solute movement and the number of ions that are coupled. The two main Na/glucose transporters are SGLT1 and SGLT2. Both belong to the SLC5 family. Although they are structurally similar, they differ in an important way. For SGLT1 two Na+ ions are coupled to the transport of each glucose molecule and for SGLT2 only one Na+ ion is coupled to the movement of each glucose molecule (Figure 3).

Figure 3 The SGLT1 and SGLT2 transporters differ in the number of sodium ions coupled to the transport of one glucose molecule.
Coupled solute movement mediated by Na/glucose transporters can be described as follows,
\[\text{glucos}\text{e}_{\text{out}}\text{\ +\ }\text{n⋅N}\text{a}_{\text{out}}^{\text{+}}\text{\ ⇌\ }\text{glucos}\text{e}_{\text{in}}\text{\ +\ }\text{n⋅N}\text{a}_{\text{in}}^{\text{+}}\]
where, n=2 for SGLT1, and n=1 for SGLT2.
For this reaction to proceed in the forward direction the electrochemical potential difference for sodium ions \((\Delta{\widetilde{\mu}}_{\mathit{Na}})\), which was calculated above, has to be greater than the chemical potential difference for glucose \({(\Delta\mu}_{\text{glucose}})\).
\[\Delta{\widetilde{\mu}}_{\mathit{Na}} > {\Delta\mu}_{\text{glucose}}\]
\[12,512\ \text{J}\text{⋅}\text{mo}\text{l}^{\text{-1}} > {\Delta\mu}_{\text{glucose}}\]
The chemical potential for glucose is given by,
\[{\Delta\mu}_{\text{glucose}} = \text{RT\ ln}\frac{\left\lbrack \text{glucose} \right\rbrack_{\text{in}}}{\left\lbrack \text{glucose} \right\rbrack_{\text{out}}}\]
Substituting,
\[12,512 > 8.314 \cdot 310 \cdot \text{ln}\left( \frac{\left\lbrack \text{glucose} \right\rbrack_{\text{in}}}{\left\lbrack \text{glucose} \right\rbrack_{\text{out}}} \right)\]
Rearranging,
\[\frac{\left\lbrack \text{glucose} \right\rbrack_{\text{in}}}{\left\lbrack \text{glucose} \right\rbrack_{\text{out}}} < e^{\left( 12,512\text{/}\left( 8.314 \cdot 310 \right) \right)}\]
Transportation will proceed in the forward direction as long as,
\[\frac{\left\lbrack \text{glucose} \right\rbrack_{\text{in}}}{\left\lbrack \text{glucose} \right\rbrack_{\text{out}}} < \ 128\]
A safety margin is required so a safe ratio for a glucose gradient to avoid reversal of the transporter will be somewhat lower than this value.
The SGLT1 transporter, which couples two sodium ions, can transport glucose up a much steeper concentration gradient (up to approximately 16,400-fold) before reversing. The metabolic cost for transport of each glucose molecule is, however, now doubled.
Both SGLT1 and SGLT2 are expressed in the kidney. The SGLT2 transporter is expressed in the early proximal tubule, where the glucose concentration in the tubule is relatively high, and the SGLT1 is located in the late proximal tubule, where the concentration of glucose is much lower. By expressing the transporters in different regions of the tubule the kidney minimizes the metabolic cost of pumping glucose while still achieving maximal recovery and limiting the risk of transporter reversal.
Na/Ca Exchanger
Secondary active transport by the Na/Ca exchanger (NCX) stoichiometrically couples the movement of three sodium ions and a single calcium ion across the membrane.
\[\text{C}\text{a}_{\text{in}}^{\text{2+}}\text{\ +\ 3N}\text{a}_{\text{out}}^{\text{+}}\text{\ ⇌\ }\text{C}\text{a}_{\text{out}}^{\text{2+}}\text{\ +\ 3}\text{N}\text{a}_{\text{in}}^{\text{+}}\text{ }\]
Three sodium ions cross in one direction for one calcium ion crossing in the opposite direction. The reaction is reversible and will proceed in the forward direction if,
\[\Delta{\widetilde{\mu}}_{\text{Ca}}\ < 3\Delta{\widetilde{\mu}}_{\mathit{Na}}\]
The electrochemical potential difference for this exchange reaction is given by,
\[\Delta{\widetilde{\mu}}_{\text{ex}} = 3\Delta{\widetilde{\mu}}_{\mathit{Na}} - \Delta{\widetilde{\mu}}_{\text{Ca}}\]
At a typical resting membrane potential and internal calcium concentration this reaction is close to equilibrium (Table 2).
Table 2 Electrochemical potential difference for the Na/Ca exchange reaction.
| [Ca2+]in | Vm (mV) | Δμ̃ex (kJ⋅mol-1) | Condition |
|---|---|---|---|
| 100 nM | -72 | -0.005 | resting state |
| 1 uM | -72 | -5.9 | |
| 10 uM | -72 | -11.9 | |
| 100 nM | 0 | 6.9 | |
| 1 uM | 0 | 1.0 | |
| 10 uM | 0 | -4.9 |
For [Ca2+]out = 1.2 mM, [Na+]out = 140 mM, [Na+]in = 15 mM, at 37°C.
If the calcium concentration inside the cell is increased without a change in membrane potential, transport of calcium out of the cell becomes increasingly favored (Δμ̃ex becomes more negative). In contrast, depolarization of the membrane without an increase in internal calcium concentration will result in reversal of the exchanger (Δμ̃ex becomes positive) and calcium ions will flow into the cell, a generally unfavorable outcome.
In neurons and muscle cells both internal calcium ion concentration and membrane potential change significantly during an action potential. Both these quantities strongly affect the electrochemical potential difference for exchange and the behavior of the Na/Ca exchanger during an action potential can be quite complex (Figure 4).
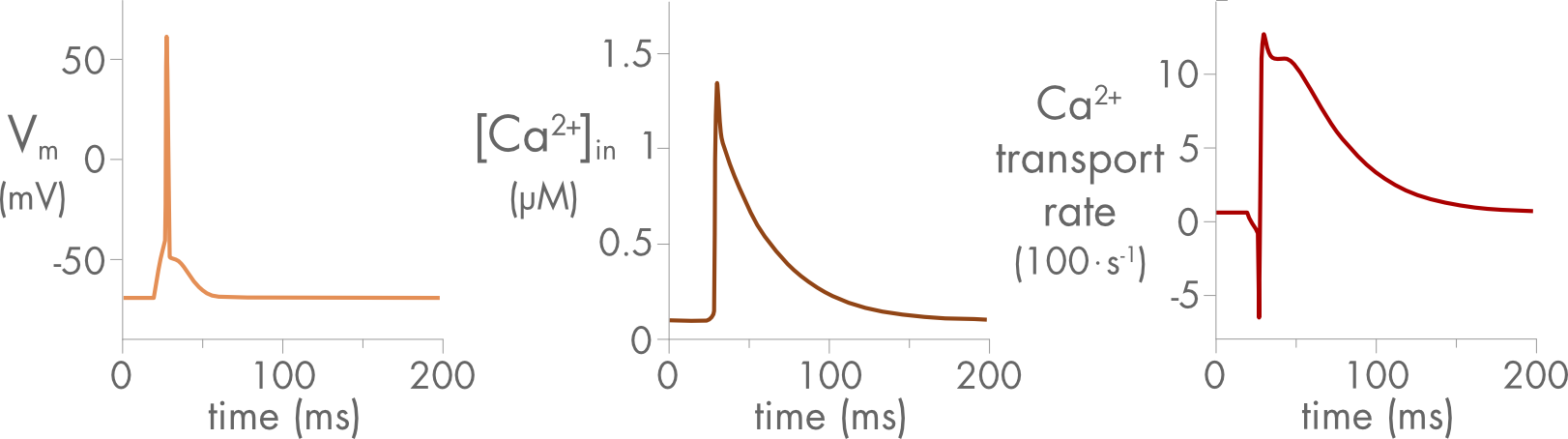
Figure 4 Changes in membrane potential, internal calcium concentration, and the calcium ion transport rate for the Na/Ca exchanger before, during and after a neuronal action potential. Initially, at rest, the exchanger is close to equilibrium and there is very little calcium ion transport. Then, during the action potential, transport turns briefly inward before becoming outward after the internal calcium ion concentration rises. The rate of transport returns to baseline in parallel with the decline in internal calcium ion concentration. Figure adapted from PhD Thesis by F. Erler, Technische Universität Dresden (2005).
Depolarization of the neuronal cell membrane during an action potential is usually accompanied by an increase in calcium as voltage gated calcium channels open. Reversal of the Na/Ca exchanger during depolarization can by itself contribute to an influx of calcium ions during an action potential, although usually much less than the flux through the channels. Typically, the exchanger will reverse during the upstroke of the action potential and then, after repolarization, primarily transport calcium ions out of the cell (Figure 4).
Although the reversal of the Na/Ca exchanger creates some risk for the cell, the transporter is valuable because it has a high turnover rate (up to several thousand calcium ions per second) and it is usually the fastest mechanism available to move calcium ions back out of the cell.