Diffusion

Thermal Energy and Brownian Motion

After leading a relatively quiet life while in crystal form, ions and other solutes that dissolve in water are then thrust into a life of constant motion. They are buffeted continuously by the movement of water molecules, which are in constant motion due to their thermal energy (Figure 1). The constant buffeting of solute molecules produces a form of movement known as Brownian motion, which can be described mathematically as a random walk.

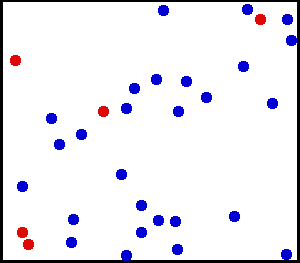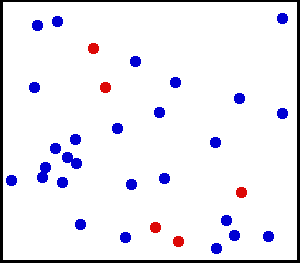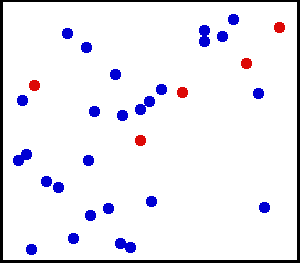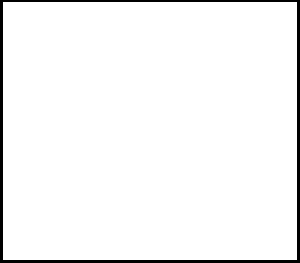
Figure 1 Movement of solute molecules (red) in water (blue). Individual molecules or ions are in constant motion due to the thermal energy of the system. Each molecule follows a random walk through space.

Diffusion of Solutes

Movement of solutes throughout the body is achieved by either bulk flow or diffusion. Bulk flow is created by pressure gradients that are generated by the heart i.e. blood flow. Bulk flow can move solutes over large distances within the body. Diffusion can only move solutes over relatively short distances with any efficiency. This has some similarities to the ‘last mile’ problem that Amazon faces for package deliveries. It is relatively inexpensive to build the infrastructure (vascular system) necessary to bring the solute close to its final destination but very expensive to deliver directly to every cell. The ‘last mile’ in the movement of solutes from the blood stream to the interior of individual cells is largely achieved by diffusion, which is sufficiently efficient over short distances.

Diffusion is a subtle phenomenon that deserves some attention because its properties have dictated much of the physical structure of cells and tissues, including the nervous system. The energy for solute movement during diffusion derives from the kinetic energy of water molecules but the direction of movement depends on the spatial distribution of solute molecules.

Figure 2 illustrates some properties of diffusion. Diffusion of a small fraction of solute molecules can be very fast over short distances, but it can be very slow to reach equilibrium. Over short distances (< 50 nm) diffusion of signaling molecules is a reasonably efficient way for cells to communicate, either intracellularly, in the case of calcium ions, or between cells, in the case of neurotransmitters. In Figure 2, once the membrane becomes permeable to the solute (at time = 75 μs), some solute molecules move very quickly into the second compartment, but it takes a considerably longer time for the two compartments to reach equilibrium. Calcium signaling in the presynaptic nerve terminal takes advantage of this behavior. When an action potential invades the nerve terminal there is a brief increase in membrane permeability to calcium ions. This allows a relatively small number of calcium ions outside the cell to rapidly enter and trigger neurotransmitter release. The membrane permeability to calcium ions is quickly terminated, however, well before the calcium concentration inside the cell reaches levels found in the extracellular medium, which would be harmful to the cell.

Figure 2 Diffusion of a solute between two compartments separated by a membrane. Initially the solute is restricted to one compartment, which is separated from the other compartment by a membrane that is impermeable to the solute. At time = 75 μs, the membrane separating the two compartments becomes permeable to the solute and solute molecules begin to diffuse into the second compartment. Entropy in the system begins to rise rapidly at this point.

A second key point illustrated in the simulation is that the separation of solutes such as sodium ions across the cell membrane, as seen in Figure 2 (at time zero), is a way to store energy to perform work. At time zero the system can perform work by coupling the flux of the solute down its concentration gradient to the transport of a different solute up its concentration, as happens in active transport. As the entropy of the system increases the ability to perform this work decreases. This is a simple illustration of Clausius’ description of entropy as that part of the energy which cannot be converted into work.

There is a constant flux of energy into biological systems that is used to locally decrease entropy in the cell, in part by creating gradients of ions and solutes across the cell membrane.